You use ANOVA: Two-Factor without Replication when each cell only has 1 observation, but use Two-Factor with Replication when each cell has more than 1 observation.
For instance, there are two factors, City and Chain Brand. Each has 2 levels, leading to 4 cells (see the figure below).
Each cell in the left-hand data table has 5 observations, and thus we use ANOVA: Two-Factor with Replication.
In contrast, each cell in the right-hand data table has only 1 observation, and thus we use ANOVA: Two-Factor without Replication.

ANOVA Two-Factor with Replication and without Replication: Difference in Variance Partitioning
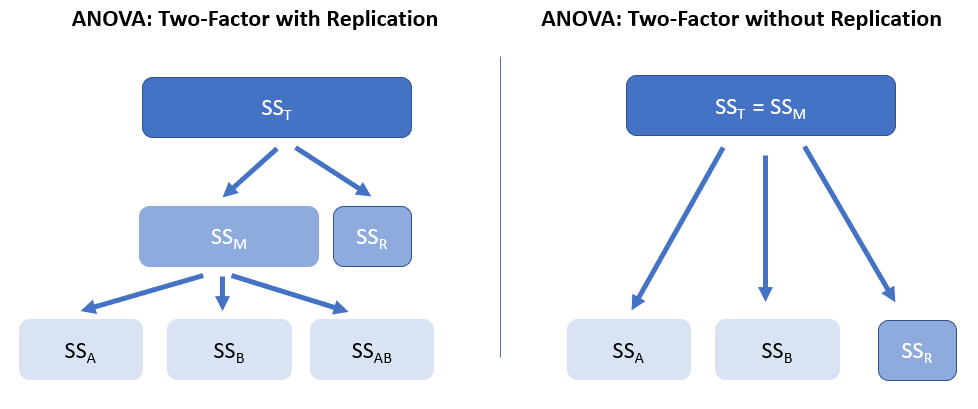
The following figure shows the difference in variance partitioning. In particular,
- ANOVA with Replication: SST is divided into SSM and SSR, and there is an interaction term SSAB.
- ANOVA without Replication: SST is equal to SSM, and there is no interaction term SSAB.
Reasons for No Interaction Term SSAB
There are two perspective to explain why there is no interaction term SSAB for ANOVA: Two-Factor Without Replication.
First, since each cell only one observation, \( x_{ij} \) is exactly the same as \( \bar{x_{ij}}\). Thus, you can not use the the same formula for SSR in two-factor ANOVA with replication. Instead, the formula for SSR for ANOVA two-Factor without Replication is as follows.
\( SS_R = \sum \sum (x_{ij}-\bar{x_i}-\bar{x_j}+\bar{x})^2 \)
where,
- \( x_{ij} \) represents observation at \( i^{th} \) row and \( j^{th} \) column.
- \( \bar{x_i}\) is the mean of observations for \( i^{th} \) row.
- \( \bar{x_j}\) is the mean of observations for \( j^{th} \) row.
- \( \bar{x}\) is the grand mean of all the cells.
Second, we can consider it from the degree of freedom perspective. The total degree of freedom is n-1 for SST. For instance, if you have 4 cells, dfT=3. Then, there is 1 df for SSA, and 1 df for SSB. Finally, there is only 1 df is left for SSR.
Further Reading
The following is the tutorial showing formulas for ANOVA Two-Factor without Replication and how to calculate it by hand and in Excel.
Further, there are related tutorials on this site on Two-factor ANOVA with replication.
Finally, there are two tutorials outside of this site as well.
